Posts filed under Denominator? (87)

From the Herald

Obviously there isn’t research giving ‘the exact time you will crash your car’.  What you might hope for is the time at which you (more precisely, the average NZ driver) are at highest risk.  We don’t even get that.

The comparisons are for totals, and as the story admits, more crashes happen in peak times because more people are driving.  It’s worse than that, though. The story says

…22,000 collisions occur annually in the afternoon peak up to 6pm. This then drops to just 2000 crashes a year at 11pm and a mere 800 at 1am.

The 22,000 is over 3-hour periods and I think the 2000 and 800 are for single-hour periods — I can’t tell for sure, because there’s no link to the original source, and I can’t find it on the IAG website.

Perhaps more relevantly for the New Zealand Herald, you have to read down to paragraph 11, which begins “Across most states…” to get the first solid indication that this story is about another country.

It’s from news.com.au, which explains why the handling of numbers isn’t up to local standards.

The World Economic Forum (the people who run the Davos meetings) are circulating this graph:

According to the graph, New Zealand is at the bottom of the OECD, with 0% waste composted or recycled.  We’ve seen this graph before, with a different colour scheme. The figure for NZ is, of course, utterly bogus.

The only figure the OECD report had on New Zealand was for landfill waste, so obviously landfill waste was 100% of that figure, and other sources were 0%.   If that’s the data you have available, NZ should just be left out of the graph — and one might have hoped the World Economic Forum had enough basic cluefulness to do so.

A more interesting question is what the denominator should be. The definition the OECD was going for was all waste sent for disposal from homes and from small businesses that used the same disposal systems as homes. That’s a reasonable compromise, but it’s not ideal. For example, it excludes composting at home. It also counts reuse and reduced use of recyclable or compostable materials as bad rather than good.

But if we’re trying to approximate the OECD definition, roughly where should NZ be?  I can’t find figures for the whole country, but there’s some relevant –if outdated — information in Chapter 3 of the Waste Assessement for the Auckland Council Waste Management Plan. If you count just kerbside recycling pickup as a fraction of kerbside recycling+waste pickup, the diversion figure is 35%. That doesn’t count composting, and it’s from 2007-8, so it’s an underestimate. Based on this, NZ is probably between USA and Australia on the graph.

Mark Hanna submitted an OIA request about strip searches in NZ prisons, which carried out with ‘reasonable grounds to believe’ the prisoner has an unauthorised item.  You can see the full response at FYI. He commented that 99.3% of these searches find nothing.

Here’s the monthly data over time:

The positive predictive value of having ‘reasonable grounds’  is increasing, and is up to about 1.5% now. That’s still pretty low. How ‘reasonable’ it is depends on what proportion of the time people who aren’t searched have unauthorised items: if that were, say, 1 in 1000, having ‘reasonable grounds’ would be increasing it 5-15-fold, which might conceivably count as reasonable.

We can look at the number of searches conducted, to see if that tells us anything about trends

Again, there’s a little good news: the number of strip searches has fallen over the the past couple of years. That’s a real rise and fall — the prison population has been much more stable. The trend looks very much like the first trend upside down.

Here’s the trend for number (not proportion) of searches finding something

It’s pretty much constant over time.

Statistical models confirm what the pictures suggest: the number of successful searches is essentially uncorrelated with the total number of searches. This is also basically good news (for the future, if not the past): it suggests that a further reduction in strip searches may well be possible at no extra risk.

Adam Feldman runs the blog Empirical SCOTUS, with analyses of data on the Supreme Court of the United States. He has a recent post (via Mother Jones) showing how often each judge was interrupted by other judges last year:

For those of you who don’t follow this in detail, Elena Kagan and Sonia Sotomayor are women.

Looking at the other end of the graph, though, shows something that hasn’t been taken into account. Clarence Thomas wasn’t interrupted at all. That’s not primarily because he’s a man; it’s primarily because he almost never says anything.

Interpreting the interruptions really needs some denominator. Fortunately, we have denominators. Adam Feldman wrote another post about them.

Here’s the number interruptions per 1000 words, with the judges sorted in order of  how much they speak

And here’s the same thing with interruption per 100 ‘utterances’

It’s still pretty clear that the female judges are interrupted more often (yes, this is statistically significant (though not very)). Taking the amount of speech into account makes the differences smaller, but, interestingly, also shows that Ruth Bader Ginsburg is interrupted relatively often.

Denominators do matter.

Two maps via Twitter:

From the Sydney Morning Herald, via @mlle_elle and @rpy

The differences in population density swamp anything else. For the map to be useful we’d need a comparison between ‘creative professionals’ and ‘non-creative unprofessionals’.  There’s an XKCD about this.

Peter Ellis has another visualisation of the last election that emphasises comparisons. Here’s a comparison of Green and Labour votes (by polling place) across Auckland.

There’s a clear division between the areas where Labour and Green polled about the same, and those where Labour did much better

The Icelandic Ethical Humanist Association commissioned a survey on religion. For people who don’t want to read the survey report (in PDF, in Icelandic), there’s a story at Iceland Magazine. The main point is in the headline: 0.0% of Icelanders 25 years or younger believe God created the world, new poll reveals.

That’s a pretty strong claim, so what did the survey actually do? Well, here you do need to read the survey report (or at least feed snippets of it to Google Translate). Of the people they sampled, 109 were in the lowest age category, which is ‘younger than 25’.  None of the 109  reported believing “God created the world” vs “The world was created in the Big Bang”.

Now, that’s not a completely clean pair of alternatives, since a fair number of people — the Pope, for example — say they believe both, but it’s still informative to some extent. So what can we say about sampling uncertainty?

A handy trick for situations like this one is the ‘rule of 3’.  If you ask N people and none of them is a creationist, a 95% confidence upper bound for the population proportion is 3/N. So, “fewer than 3% of Icelanders under 25 believe God created the world”

It was front page news in the Dominion Post today that about 0.1% of registered teachers had been investigated for “possible misconduct or incompetence in which their psychological state may have been a factor.”  Over a six year period. And 5% of them (that is, 0.005% of all teachers) were struck off or suspended as a result.

Actually, the front page news was even worse than that:

but since the “mentally-ill” bit wasn’t even true, the online version has been edited.

Given the high prevalence of some of these psychological and neurological conditions and the lack of a comparison group, it’s not even clear that they increase the risk of being investigated or struck off . After all, an early StatsChat story was about a Dom Post claim that “hundreds of unfit teachers” were working in our schools, based on 664 complaints over two years.

It would be interesting to compare figures for, say, rugby players or journalists. Except that would be missing the other point.  As Jess McAllen writes at The Spinoff, the phrasing and placement of the story, especially the original one, is a clear message to anyone with depression, or anxiety, or ADHD. Anyone who wants to think about the children might think about what that message does for rather more than 0.1% of them.

(via @publicaddress)

Stuff has a story under the headline ACC statistics show New Zealand’s riskiest industries. They don’t. They show the industries with the largest numbers of claims.

To see why that’s a problem, consider instead the number of claims by broad ethnicity grouping: 135000 for European, 23100 for Māori, 10800 for Pacific peoples(via StatsNZ). There’s no way that European ethnicity gives you a hugely greater risk of occupational injury than Māori or Pacific workers have. The difference between these groups is basically just population size. The true risks go in the opposite direction: 89 claims per 1000 full-time equivalent workers of European ethnicities, 97 for Māori, and 106 for Pacific.

With just the total claims we can’t tell whether working in supermarkets and grocery stores is really much more dangerous than logging, as the story suggests. I’m dubious, but.

There was a lot of coverage yesterday about a potential new test for pancreatic cancer. 3News covered it, as did One News (but I don’t have a link). There’s a detailed report in the Guardian, which starts out:

A simple urine test that could help detect early-stage pancreatic cancer, potentially saving hundreds of lives, has been developed by scientists.

Researchers say they have identified three proteins which give an early warning of the disease, with more than 90% accuracy.

This is progress; pancreatic cancer is one of the diseases where there genuinely is a good prospect that early detection could improve treatment. The 90% accuracy, though, doesn’t mean what you probably think it means.

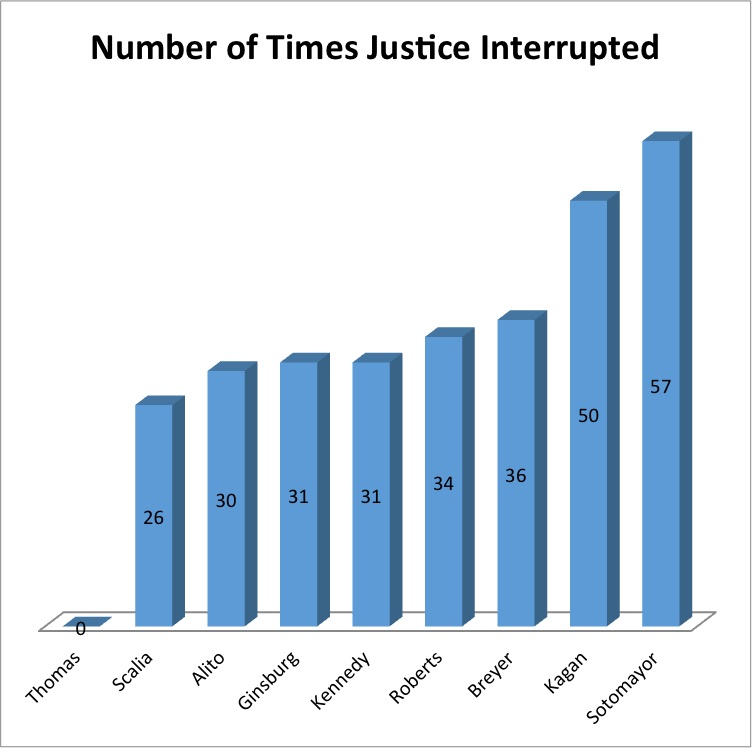
Here’s a graph showing how the error rate of the test changes with the numerical threshold used for diagnosis (figure 4, panel B, from the research paper)

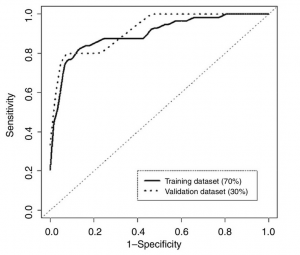
As you move from left to right the threshold decreases; the test is more sensitive (picks up more of the true cases), but less specific (diagnoses more people who really don’t have cancer). The area under this curve is a simple summary of test accuracy, and that’s where the 90% number came from.  At what the researchers decided was the optimal threshold, the test correctly reported 82% of early-stage pancreatic cancers, but falsely reported a positive result in 11% of healthy subjects.  These figures are from the set of people whose data was used in putting the test together; in a new set of people (“validation dataset”) the error rate was very slightly worse.

The research was done with an approximately equal number of healthy people and people with early-stage pancreatic cancer. They did it that way because that gives the most information about the test for given number of people.  It’s reasonable to hope that the area under the curve, and the sensitivity and specificity of the test will be the same in the general population. Even so, the accuracy (in the non-technical meaning of the word) won’t be.

When you give this test to people in the general population, nearly all of them will not have pancreatic cancer. I don’t have NZ data, but in the UK the current annual rate of new cases goes from 4 people out of 100,000 at age 40 to 100 out of 100,000 people 85+.   The average over all ages is 13 cases per 100,000 people per year.

If 100,000 people are given the test and 13 have early-stage pancreatic cancer, about 10 or 11 of the 13 cases will have positive tests, but so will 11,000 healthy people.  Of those who test positive, 99.9% will not have pancreatic cancer.  This might still be useful, but it’s not what most people would think of as 90% accuracy.

A year-old pie chart describing Google+ users. On the right are two slices that would make up a valid but pointless pie chart: their denominator is Google+ users. On the left, two slices that have completely different denominators: all marketers and all Fortune Global 100 companies.

On top of that, it’s unlikely that the yellow slice is correct, since it’s not clear what the relevant denominator even is. And, of course, though most of the marketers probably identify as male or female, it’s not clear how the Fortune Global 100 Companies would report their gender.

From @NoahSlater, via @LewSOS, originally from kwikturnmedia about 18 months ago.